Free Articles: Elementary theory of the reals

Real closed field

In mathematics, a real closed field is a field F that has the same first-order properties as the field of real numbers. Some examples are the field of real numbers, the field of real algebraic numbers, and the field of hyperreal numbers.

Definitions

A real closed field is a field F in which any of the following equivalent conditions are true:

F is elementarily equivalent to the real numbers. In other words it has the same first-order properties as the reals: any sentence in the first-order language of fields is true in F if and only if it is true in the reals.
There is a total order on F making it an ordered field such that, in this ordering, every positive element of F is a square in F and any polynomial of odd degree with coefficients in F has at least one root in F.
F is a formally real field such that every polynomial of odd degree with coefficients in F has at least one root in F, and for every element a of F there is b in F such that a = b or a = −b.
F is not algebraically closed but its algebraic closure is a finite extension.
F is not algebraically closed but the field extension $F(\sqrt{-1})$ is algebraically closed.
There is an ordering on F which does not extend to an ordering on any proper algebraic extension of F.
F is a formally real field such that no proper algebraic extension of F is formally real. (In other words, the field is maximal in an algebraic closure with respect to the property of being formally real.)
There is an ordering on F making it an ordered field such that, in this ordering, the intermediate value theorem holds for all polynomials over F.
F is a field and a real closed ring.

If F is an ordered field (not just orderable, but a definite ordering is fixed as part of the structure), the Artin–Schreier theorem states that F has an algebraic extension, called the real closure K of F, such that K is a real closed field whose ordering is an extension of the given ordering on F, and is unique up to a unique isomorphism of fields (note that every ring homomorphism between real closed fields automatically is order preserving, because x ≤ y if and only if ∃z y = x+z). For example, the real closure of the rational numbers is the field $\mathbb{R}_{alg}$ of real algebraic numbers. The theorem is named for Emil Artin and Otto Schreier, who proved it in 1926.

If F is a field (so this time, no order is fixed, and it is even not necessary to assume that F is orderable) then F still has a real closure, which in general is not a field anymore, but a real closed ring. For example the real closure of the field $\mathbb{Q}(\sqrt 2)$ is the ring $\mathbb{R}_{alg}\times \mathbb{R}_{alg}$ (the two copies correspond to the two orderings of $\mathbb{Q}(\sqrt 2)$ ). Whereas the real closure of the ordered subfield $\mathbb{Q}(\sqrt 2)$ of $\mathbb{R}$ is again the field $\mathbb{R}_{alg}$ .

Model Theory: decidability and quantifier elimination

The theory of real closed fields was invented by algebraists, but taken up with enthusiasm by logicians. By adding to the ordered field axioms

one obtains a first-order theory. Tarski (1951) proved that the theory of real closed fields in the first order language of partially-ordered rings (consisting of the binary predicate symbols "=" and "≤", the operations of addition, subtraction and multiplication and the constant symbols 0,1) admits elimination of quantifiers. The most important model theoretic consequences hereof: The theory of real closed fields is complete, o-minimal and decidable.

Decidability means that there exists at least one decision procedure, i.e., a well-defined algorithm for determining whether a sentence in the first order language of real closed fields is true. Euclidean geometry (without the ability to measure angles) is also a model of the real field axioms, and thus is also decidable.

The decision procedures are not necessarily practical. The algorithmic complexities of all known decision procedures for real closed fields are very high, so that practical execution times can be prohibitively high except for very simple problems.

The algorithm Tarski proposed for quantifier elimination has NONELEMENTARY complexity, meaning that no tower $2^{2^{\cdot^{\cdot^{\cdot^n}}}}$ can bound the execution time of the algorithm if n is the size of the problem. Davenport and Heintz (1988) proved that quantifier elimination is in fact (at least) doubly exponential: there exists a family Φ_n of formulas with n quantifiers, of length O(n) and constant degree such that any quantifier-free formula equivalent to Φ_n must involve polynomials of degree $2^{2^{\Omega(n)}}$ and length $2^{2^{\Omega(n)}}$ , using the Ω asymptotic notation.

Basu and Roy (1996) proved that there exists a well-behaved algorithm to decide the truth of a formula ∃x₁,…,∃x_k P₁(x₁,…,x_k)⋈0∧…∧P_s(x₁,…,x_k)⋈0 where ⋈ is <, > or =, with complexity in arithmetic operations sd.

Order properties

A crucially important property of the real numbers is that it is an archimedean field, meaning it has the archimedean property that for any real number, there is an integer larger than it in absolute value. An equivalent statement is that for any real number, there are integers both larger and smaller. A non-archimedean field is, of course, a field that is not archimedean, and there are real closed non-archimedean fields; for example any field of hyperreal numbers is real closed and non-archimedean.

The archimedean property is related to the concept of cofinality. A set X contained in an ordered set F is cofinal in F if for every y in F there is an x in X such that y < x. In other words, X is an unbounded sequence in F. The cofinality of F is the size of the smallest cofinal set, which is to say, the size of the smallest cardinality giving an unbounded sequence. For example natural numbers are cofinal in the reals, and the cofinality of the reals is therefore $\aleph_0$ .

We have therefore the following invariants defining the nature of a real closed field F:

To this we may add

These three cardinal numbers tell us much about the order properties of any real closed field, though it may be difficult to discover what they are, especially if we are not willing to invoke generalized continuum hypothesis. There are also particular properties which may or may not hold:

The generalized continuum hypothesis

The characteristics of real closed fields become much simpler if we are willing to assume the generalized continuum hypothesis. If the continuum hypothesis holds, all real closed fields with cardinality the continuum and having the η₁ property are order isomorphic. This unique field Ϝ can be defined by means of an ultrapower, as $\Bbb{R}^{\Bbb{N}}/{\mathbf M}$ , where M is a maximal ideal not leading to a field order-isomorphic to $\Bbb{R}$ . This is the most commonly used hyperreal number field in nonstandard analysis, and its uniqueness is equivalent to the continuum hypothesis. (Even without the continuum hypothesis we have that if the cardinality of the continuum is $\aleph_\beta$ then we have a unique η_β field of size η_β.)

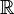
Moreover, we do not need ultrapowers to construct Ϝ, we can do so much more constructively as the subfield of series with a countable number of nonzero terms of the field $\Bbb{R}((G))$ of formal power series on the .

Ϝ however is not a complete field; if we take its completion, we end up with a field Κ of larger cardinality. Ϝ has the cardinality of the continuum which by hypothesis is $\aleph_1$ , Κ has cardinality $\aleph_2$ , and contains Ϝ as a dense subfield. It is not an ultrapower but it is a hyperreal field, and hence a suitable field for the usages of nonstandard analysis. It can be seen to be the higher-dimensional analogue of the real numbers; with cardinality $\aleph_2$ instead of $\aleph_1$ , cofinality $\aleph_1$ instead of $\aleph_0$ , and weight $\aleph_1$ instead of $\aleph_0$ , and with the η₁ property in place of the η₀ property (which merely means between any two real numbers we can find another).

Examples of real closed fields

References

Basu, Saugata, , and Marie-Françoise Roy (2003) "Algorithms in real algebraic geometry" in Algorithms and computation in mathematics. Springer. ISBN 3540330984 (online version)
Caviness, B F, and Jeremy R. Johnson, eds. (1998) Quantifier elimination and cylindrical algebraic decomposition. Springer. ISBN 3211827943
Chen Chung Chang and Howard Jerome Keisler (1989) Model Theory. North-Holland.
Dales, H. G., and W. Hugh Woodin (1996) Super-Real Fields. Oxford Univ. Press.
Mishra, Bhubaneswar (1997) "Computational Real Algebraic Geometry," in Handbook of Discrete and Computational Geometry. CRC Press. 2004 edition, p. 743. ISBN 1-58488-301-4
Alfred Tarski (1951) A Decision Method for Elementary Algebra and Geometry. Univ. of California Press.
J. Davenport and J. Heintz, "Real quantifier elimination is doubly exponential", Journal of Symbolic Computation 5:1–2 (1988), pp. 29–35.

External links

Retrieved from : http://en.wikipedia.org/wiki/Real_closed_field

2011-03-29

Elementary theory of the reals